Answer by lenny460 (1073) ( Show Source ) You can put this solution on YOUR website!Here is the question What is the coefficient of w˛xłyzł in the expansion of (wxyz) 9 There are 9 4term factors in (wxyz) 9 (wxyz)(wxyz)(wxyz)(wxyz)(wxyz)(wxyz)(wxyz)(wxyz)(wxyz) To multiply it all the way out we would choose 1 term from each factor of 4 terms To get w˛xłyzł, · (xy)^3 (yz)3 (zx)^3 = 3(xy)(yz)(zx) That is it no constraints etc It mentions "This can be done by expanding out the brackets, but there is a more elegant solution" Homework Equations The Attempt at a Solution First of all this only seems to hold in special cases as I have substituted random values for x,y and z and they do not agree

Xyz Wikipedia
What is the formula of (x+y+z)^3
What is the formula of (x+y+z)^3-Given that x 3 4y 3 9z 3 = 18xyz and x 2y 3z = 0 x 2y = 3z, 2y 3z = x and 3z x = 2y Now `( x 2y )^2/(xy) (2y 3z)^2/(yz) (3x x)^2/(zx · 8x y z = 9, 2x 2y 3z = 22 and x 3y 2z = 15 solve for an ordered tripple asked Mar 6, 14 in ALGEBRA 2 by harvy0496 Apprentice systemofequations



Case Study Xyz Bank Ppt Powerpoint Presentation Pictures Guide Graphics Presentation Background For Powerpoint Ppt Designs Slide Designs
3 The right hand and left hand limit of the function are respectively 4 If tan A cot A = 2, then the value of t a n 4 A c o t 4 A = 5 If y = 2 x n 1 3 x n ,then x 2 d 2 y d x 2 is 6 If the curves 2 x = y 2 and 2 x y = K intersect perpendicularly, then the value of K 2 isThe author is saying y is not a particular number, that equation holds for every real number So it works for y=3 but also for y = 3 or y = \pi or whateverExpand (xy)^3 full pad » x^2 x^ {\msquare} \log_ {\msquare} \sqrt {\square} \nthroot \msquare {\square} \le \ge
This is the Solution of Question From RD SHARMA book of CLASS 9 CHAPTER POLYNOMIALS This Question is also available in R S AGGARWAL book of CLASS 9 You can FThis preview shows page 3 6 out of 11 pages 33 Find the sumofproducts expansion of the Boolean function f (x y z) that is 1 if and only if exactly two of the three variables have value 1 Ans xyz xyz xyz 34 Find the sumofproducts expansion of the Boolean function f (x y z) that is 1 if and only if either x z 1 and y 0, or x 0 and y z 1This term will be of the form n x 3 y 2 z 3 w for some integer n, so its "coefficient" is n w We can expand ( 2 x 3 y − 4 z w) 9 = ( ( 2 x 3 y − 4 z) w) 9 as ( 2 x 3 y − 4 z) 9 9 w ( 2 x 3 y − 4 z) 8 , so n is equal to 9 times the coefficient of x 3 y 2 z 3 in the expansion of ( 2 x 3 y − 4 z
As you can see for (a b)n contains just n 1 terms Note that we have to keep the sum of powers in each of the combinations of x, y, z to n, so it will be reduced Now replace a and b by x and (y z) respectively So total number of terms should be 1 2 3 ⋯ (n 1) = (n 1)(n 2) 2 Share · Explanation (x −y)3 = (x − y)(x −y)(x −y) Expand the first two brackets (x −y)(x − y) = x2 −xy −xy y2 ⇒ x2 y2 − 2xy Multiply the result by the last two brackets (x2 y2 −2xy)(x − y) = x3 − x2y xy2 − y3 −2x2y 2xy2 ⇒ x3 −y3 − 3x2y 3xy2Obtain The Taylor Expansion Of Z(x, Y) Near (0,0) Upto The Second Order Using The Gradient And Hessian Matrix Z(x, Y) Is Given In Implicit Form As F(x, Y, Z) = Z Cos X X2 – Eyz = 0 This problem has been solved!



How To Simplify Math X Y Z 2 X Y Z 2 X Y Z 2 X Y Z 2 Math Quora



Top 10 Strongest Rank 10 Xyz Monsters In Yu Gi Oh Hobbylark
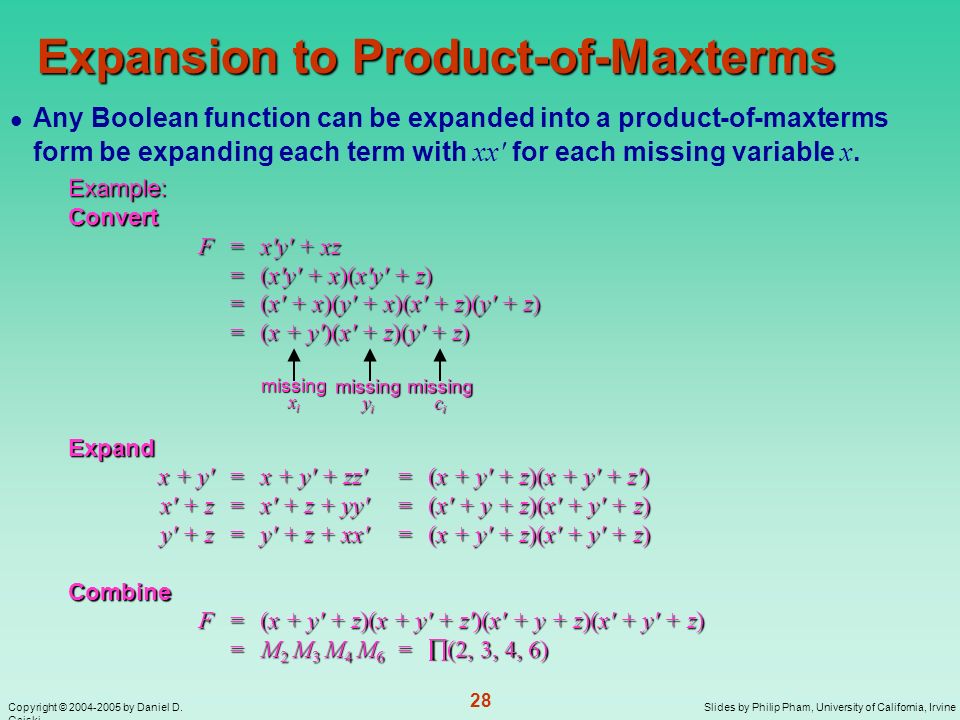
Therefore, F = m3 m4 m5 m6 m7, which is the same as above when we used term expansion x y z Minterms Notation 0 0 0 x' y' z' m0 0 0 1 x' y' z m1 0 1 0 x' y z' m2 0 1 1 x' y z m3 1 0 0 x y' z' m4 1 0 1 x y' z m5 1 1 0 x y z' m6 1 1 1 x y z m7 Table 39 F = x' y z x y' z x y z' x y z0218 · The number of terms in the expansion of (xyz) n Related questions 0 votes 1 answer If the integers r > 1, n > 2 and coefficients of (3r)th and (r 2)nd terms in the binomial expansion of (1 x)2n are equal, then asked Feb , 18 in Class XI Maths by rahul152Question from Binomial Theorem,cbse,math,class11,ch8,binomialtheorem,exemplar,q26,secb,medium


Principles Of Digital Design Chapter 3 Boolean Algebra And Logic Design Boolean Algebra Logic Gates Digital Design Implementation Technology Asics Ppt Download



Expand Expand Terms Multiply Polynomials With Step By Step Math Problem Solver
See the answer Show transcribed image text Expert AnswerC n are the binomial coefficients in the expansion of (1 x) n then prove that 2 (3 2 − 1) C 1 2 2 (3 2 2 2 − 1) C 2 2 3 (3 3 2 3 − 1) C 3 2 n (3 n 2 n − 1) C n = 2 n (2 3 n − 3 n)The binomial theorem (or binomial expansion) is a result of expanding the powers of binomials or sums of two terms The coefficients of the terms in the expansion are the binomial coefficients


Formula For X Y Z 2 Brainly In



Part 5 Xy Wings Xyz Wings By Dan Lekander Thousand Islands Life Magazine Thousand Islands Life Magazine All Archives
We know the corollary if abc = 0 then a3 b3 c3 = 3abcUsing the above identity taking a = x−y, b = y−z and c= z−x, we have abc= x−yy−zz −x= 0 then the equation (x− y)3 (y−z)3 (z−x)3 can be factorised as follows(x−y)3 (y−z)3 (z−x)3 = 3(x−y)(y−z)(z−x)Hence, (x−y)3 (y−z)3 (z −x)3 = 3(x−y)(y −z)(z −x)243x 5 810x 4 y 1080x 3 y 2 7x 2 y 3 240xy 4 32y 5 Finding the k th term Find the 9th term in the expansion of (x2y) 13 Since we start counting with 0, the 9th term is actually going to be when k=8 That is, the power on the x will 138=5 and the power on the 2y will be 8Answered 1 year ago · Author has 115 answers and 3342K answer views Solution here, = (Xyz) (Xyz)² = (Xyz) (x²xyxzyxy²yzzxzyz²) = (x³y³z³3x²y3xy²3x²z3z²x3y²z3z²y6xyz) 38K views · View upvotes



Find The Coemcient Of Xyz In The Expansion Of X Y Z Find The Coefficient Of X In The Expansion Of 1 1 X 1 X 2



Xyz Affair Wikipedia
This information can be summarized by the Binomial Theorem For any positive integer n, the expansion of (x y)n is C(n, 0)xn C(n, 1)xn1y C(n, 2)xn2y2 C(n, n 1)xyn1 C(n, n)yn Each term r in the expansion of (x y)n is given by C(n, r 1)xn (r1)yr1 Example Write out the expansion of (x y)75!) Thus, (xyz) 10 = ∑(10!) / (P1(x y) 3 = x 3 3x 2 y 3xy 2 y 3 Example (1 a 2 ) 3 = 1 3 31 2 a 2 31(a 2 ) 2 (a 2 ) 3 = 1 3a 2 3a 4 a 6 (x y z) 2 = x 2 y 2 z 2 2xy 2xz 2yz



Let X Y Z Be Real Numbers Satisfying X Y Z 3 X 2 Y 2 Z 2 5


Math In Lwarp Via Controlled Expansion Tex Latex Stack Exchange



0 件のコメント:
コメントを投稿